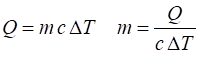|
3.2 THERMODYNAMICS P32 006 A
health clinic offers a program, weight loss with
cold water. They claim that participants, by drinking cold water,
can eat as much as they like and not gain weight. Assume
that a participant in the program had a snack consisting of a pancake (200 g)
with jam (20 g) and cream (30 g). Determine how much cold water at 0 oC must be drunk to counteract the snack. Food-Energy
Data (MJ.kg-1): pancake (17.6) jam (12.4) cream (20.0) specific
heat of water c = 4180 J.kg-1.K-1
View solution below only after you have completed answering the question. The solution is not in a form that you would answer in an examination. The answers are often in more detail to help improve your appreciation and understanding of the physics. |
|
Solution Knowledge specific heat Energy provided by snack pancake 17.6x106 J.kg-1 200x10-3 kg Epancake = (17.6x106) (200x10-3) J = 3.52x106 J jam
12.4x106 J.kg-1 20x10-3 kg Epancake = (12.4x106) (20x10-3) J = 2.48x105 J
cream 20.0x106 J.kg-1 30x10-3 kg Epancake = (20.0x106) (30x10-3) J = 6.00x105 J Total energy provided from snack Esnack = (3.52x106 + 2.48x105 + 6.00x105) J = 4.37x106 J
We need to raise the temperature of the cold water from 0 oC to 37 oC from the energy provided by the snack. Density of water = 1000 kg.m-3 = 1 kg.L-1 Therefore, you would have to drink about 28 one-litre bottles of cold water to counteract the snack. This is a lot of water.
|
|
VISUAL PHYSICS ONLINE http://www.physics.usyd.edu.au/teach_res/hsp/sp/spHome.htm If you have any feedback, comments,
suggestions or corrections please email: Ian Cooper
School of Physics University
of Sydney ian.cooper@sydney.edu.au |