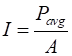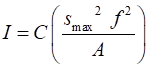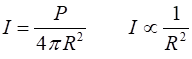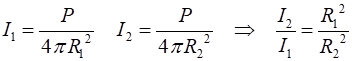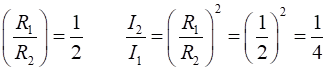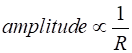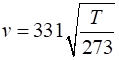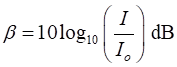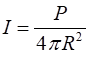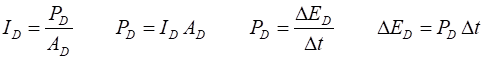Wave Intensity

WAVES

BEHAVIOUR OF WAVES

ENERGY TRANSPORTED BY WAVES

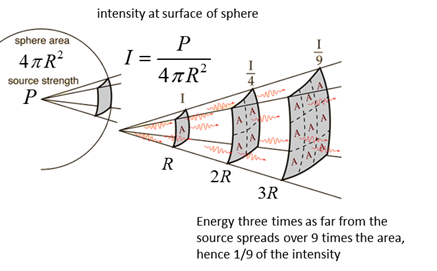
Waves transport energy from one place to another. To a good approximation, the energy transported by a wave is proportional to its square of the amplitude and to the square of its frequency . Hence, the average rate of energy transfer is the average power

The intensity if a wave is defined as the ratio of the average power to the area perpendicular to the direction of energy flow that the energy passes through

[ W.m^{-2 ]}

is a constant of proportionality

If a wave spreads out form the source in all directions, it is a three-dimensional wave. If the energy spreads uniformly in all directions in a isotropic medium (same in all directions), the wave is said to be a spherical wave. As the wave moves outward, the energy it carries is spread over a larger and larger area since the surface area of a sphere of radius is . Thus, for our spherical wave with a constant power output , its intensity is given by

inverse square law for a spherical wave

This is known as the inverse square law.

If we consider two points at distances and from the source, then

For example, if the distance doubles, then the intensity is reduced by a

The amplitude of a wave also decreases with distance. Since the intensity is proportional to the square of the amplitude, then the amplitude must be inversely proportional to the distance

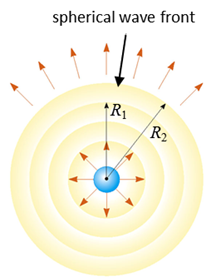
When a wave is twice as far from its source, the amplitude is half as large (spherical wave propagating in an isotropic medium).

A wavefront is a line or surface that joins points of same phase.

For water waves travelling on the surface from a point source, the wavefronts are circles. For sound waves emanating from a point source, the wavefronts are spherical surfaces. In modelling waves propagating in one-dimension, the waves are referred to as plane waves.

SPEED OF SOUND

The speed of sound is different in different materials and is very dependent on the temperature for gaseous media. The more elastic the medium the greater the speed and the greater the density the slower the speed. The speed of sound in air at a temperature [K kelvin] is approximately given by

speed of sound in air

= 0 ^oC = 273 K = 331 m.s^-1

= 20 ^oC = 293 K = 343 m.s^‑1

The dependence of the speed of sound on the air temperature leads to interesting effects on the propagation of sound due to refraction (bending of the wavefronts due to change in speed of the wave).

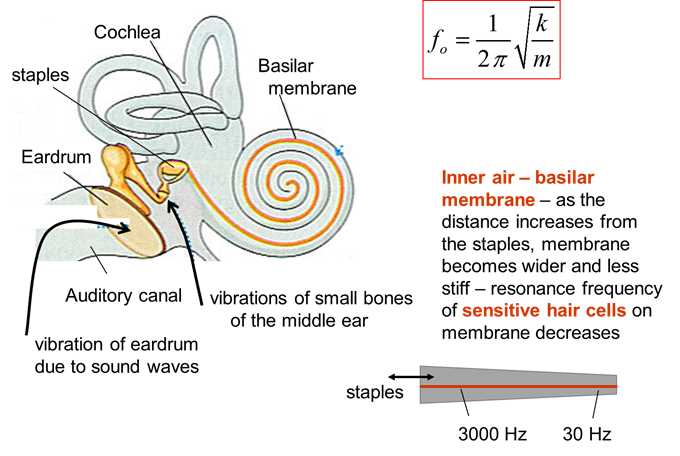
SOUNDS and HEARING

Sounds are the longitudinal waves due to the vibrations of molecules. In air, the sound that we perceive is associated with our sense of hearing and, therefore, with the physiology of our ears and the psychology of our brain.

Two aspects of any sound are its loudness and pitch. Loudness and pitch are subjective quantities and depend upon the consciousness of the listener.

Loudness is related to the energy in the sound wave and its frequency.

Pitch is related to frequency and changes in energy and refers to whether the sound is high (violin) or low (bass drum). The higher the frequency the higher the pitch.

The human ear responds to the audible range of frequencies from about 20 Hz to less than 20 kHz. As we get older, we lose the ability to hear the higher frequency components of any sound. Some people with hearing problems can only hear frequencies less than about 8 kHz.

One resonance (natural) frequency of the auditory canal is around 3000 Hz, so, sounds with component frequency near 3000 Hz are perceived to be loud than other sounds.

Sounds are longitudinal wave that can propagate through any medium which can be compressed such as a gas, liquid or solid.

In air, audible human hearing frequency range is ~20 Hz to ~ 20 kHz.

Frequencies less than 20 Hz are called infrasound. Animals have been known to perceive the infrasonic waves going through the Earth caused by natural disasters and can use these as an early warning. A recent example of this is the 2004 Indian Ocean earthquake and tsunami. Animals were reported to flee the area hours before the actual tsunami hit the shores of Asia. Infrasound is one of several techniques used to identify if a nuclear detonation has occurred.

Frequencies above 20 kHz are called ultrasound. Many animals can hear ultrasound frequencies: dogs hear sounds as high as 50 kHz and but can detect frequency as high as 100 kHz.

Small objects can be imaged using ultrasonic waves because of their short wavelengths (high frequencies). So, ultrasound waves are used widely in medicine to image internal organs and blood vessels. The images are produced by the reflection and absorption of ultrasonic waves. Use of ultrasonic waves is safer than X-rays but the images show less details. Certain organs such as the liver and the spleen are invisible to X-rays but visible to ultrasonic waves.

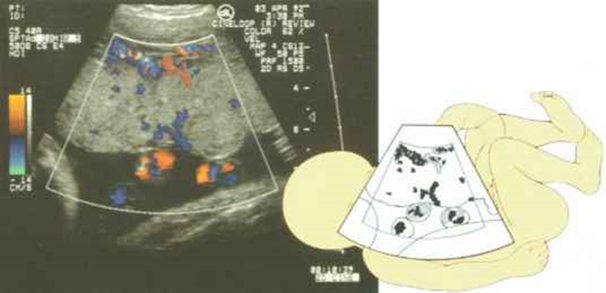
Blood flow through the placenta using utrasonic waves.

Applying the principle of the Doppler Effect, the speed

of blood flow can be estimated.

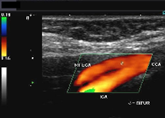
Ultrasound and color Doppler images of the normal renal arteries and renal veins.

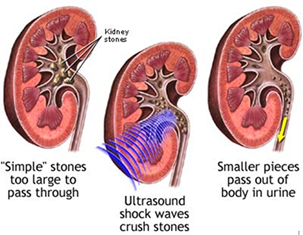
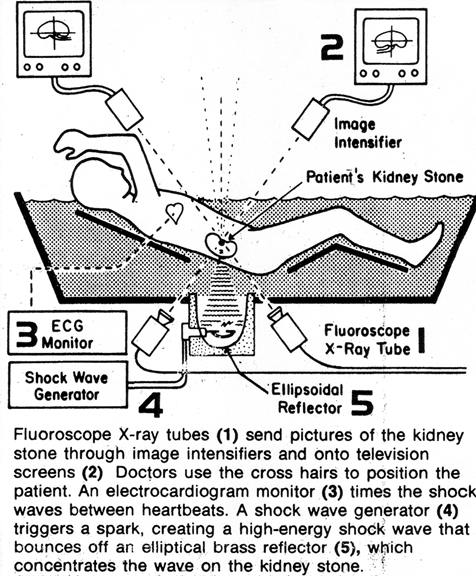
Shock waves are high energy waves. For example, high energy ultrasonic waves can be used to smash kidney stones in a procedure called extracorporeal shock wave lithotripsy.

SOUND LEVELS

A relationship between the subjective sensation loudness and the physical measurable quantity intensity can be made using a logarithmic scale called the decibel scale [dB] because the sensation of loudness is approximately logarithmic in the human ear.

Sound Level

where = 1.0x10^-12 W.m^-2 is the reference level for the sound intensity at the threshold of hearing.

Sound levels for a variety of sounds

Image result for image ears response curves

Threshold of hearing: The faintest sounds the human ear can detect at a frequency of 1 kHz have an intensity of about 1x10^-12 W.m^-2.

Threshold of pain: the loudest sounds the human ear can tolerate have an intensity of about 1 W.m^-2.

Listen to a set of pure tones (single frequency / monochromatic audio signals)

ONLINE TONE GENERATOR: play with sounds test your hearing

Example 1

A point source of sound waves emits a disturbance with a power of 50 W into a surrounding isotropic-homogeneous medium. Determine the intensity of the radiation at distances of 1.00 m, 2.00 m and 10.0 m from the source. How much energy arrives on a small detector 1.0 m from the source with an area of 123 mm² held perpendicular to the flow in 10 s?

Solution

P = 50 W

R₁ = 1.00 m I₁ = ? W.m^-2 A_D = 123 mm² = 123x10^-6 m² =10 s